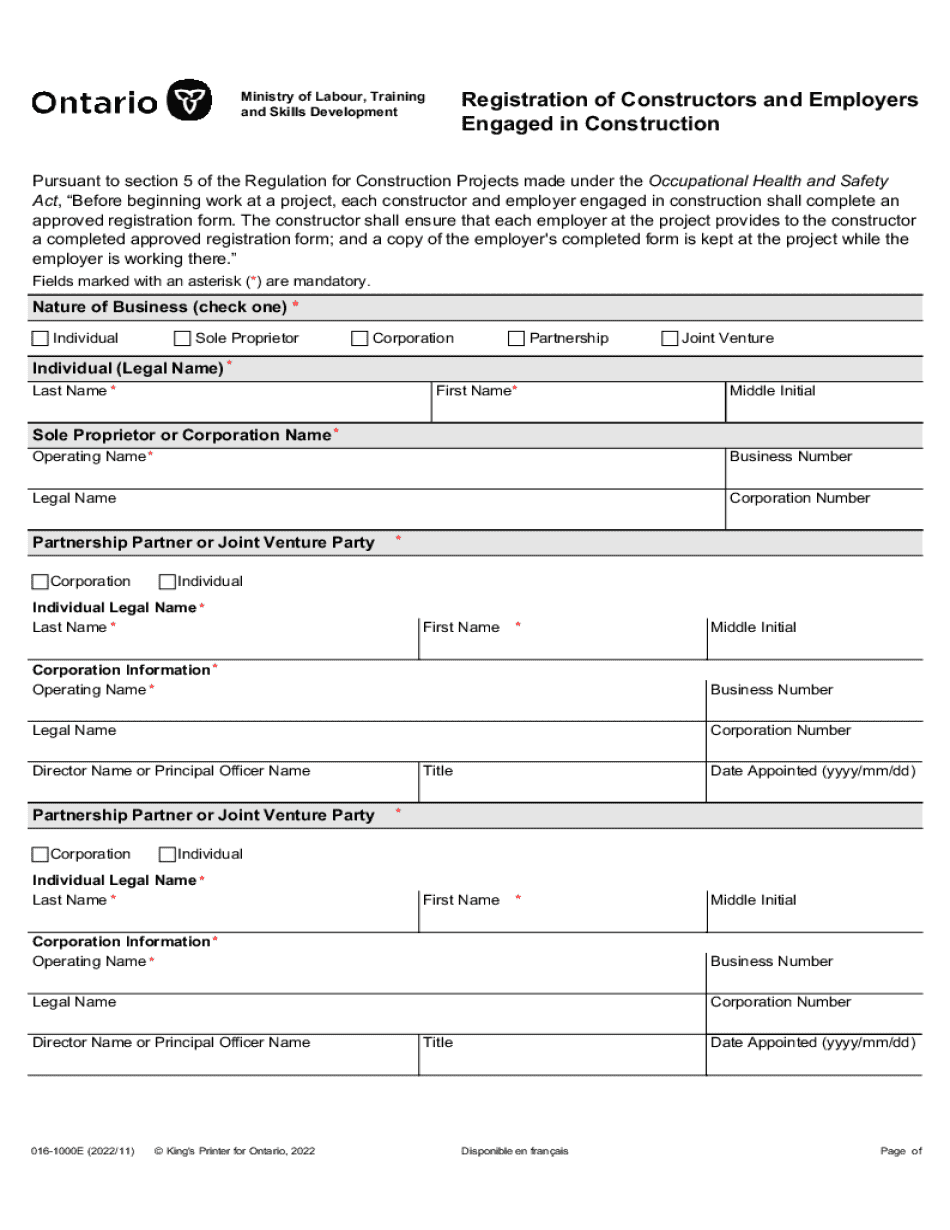
How many positive integers?
Let's first just consider solutions with b=c. Then we are looking at numbers of the form 2b(a-b)^3. If we let a=b+1 then it is obviously possible to generate all integers of the form 2b. Can we generate any odd integers? No, if we consider the expression mod 2, then (x-y)^3 \equiv (x-y), so a(b-c)^{3}+b(a-c)^{3}+c(a-b)^{3}\equiv a(b-c)+b(a-c)+c(a-b)\equiv 2ab - 2bc \equiv Thus, the given expression is always even for integer arguments. So, as David discovered, there are 500 possible values between 1 and 1000.